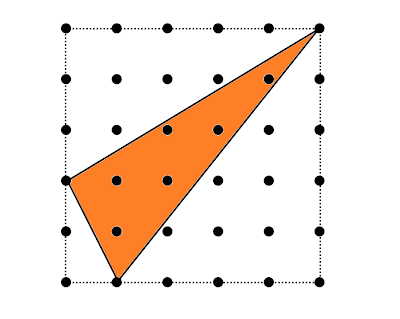
for a 5 by 5 grid
triangles are formed by joining the top right hand corner of square to two grid points (integer)
which of the four triangles looks as if it has the biggest area?
how can you work out the area of this triangle?
one of the corners must be at (5, 5) and the other two corners must lie at the 'dot' points on the x-axis and y-axis (one on each)
what whole number areas can be obtained? [there are 5 of them]
what are the smallest and largest areas that can be obtained?
establish that there are 14 possible areas
what is a general rule for the area of a triangle with coordinates at (5, 5) (x, 0) and (0, y)?
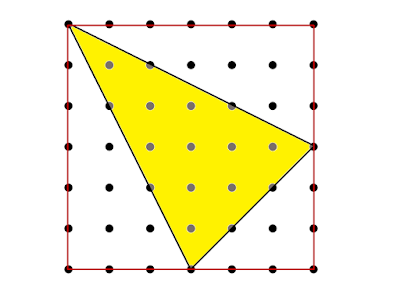
for a 6 by 6 grid
using geometry rather than numbers (or algebra)
what fraction is the triangle of the square?
many thanks to Puntmat for this insight
(Dec 7th 2015)
one way to show it










No comments:
Post a Comment