trisides

a ppt is here

a tessellation is a way of covering the plane with (usually) just one shape
repeated, regularly, without any gaps
forming a structure
'stripes' emerge

Geoff Giles (of DIME materials fame, see some of his work here) made reference to a way of creating shapes that tessellate
by modifying triangles
he called these 'trisides'

these ideas are not quite the same as Geoff's but have the same principle structure (my article was published on transforming tessellating shapes into 'trisides' in Mathematics in School, volume 14, issue 3, may 1985)

to form a 'triside' from a given (tessellating) shape:

• the routes/sections of lines, sometimes extended, between two dots (which are corners of a triangle, the 'triside') must have rotational symmetry, order 2
• all of the perimeter (boundary) length sections of the original shape must be included within the three 'triside' sections

in this way, a tessellation of a complex shape can be seen to have a tessellation of triangles 'behind it'

all quadrilaterals will tessellate

four different constructions for 'trisides' of the same quadrilateral (above) ~ two pairs of congruent triangles












how can you (easily) see that the 'trisides' (above) all have the same area? [= 18]
how can you see that this is the area of the quadrilateral
[drop a perpendicular from the top left corner]

it seems that for any quadrilateral you can start at a mid-point of any of the sides to form a 'triside'

even if it is concave

this is a general method to turn a quadrilateral (since they always tessellate) into a triangle of equal area

a triomino (a figure with 3 squares)

a quadromino

to create a 'triside' for a pentomino (all of which tessellate) usually requires going beyond the perimeter of the shape to place a dot (as a corner of a 'triside')

possibly with these 'construction' lines, the whole of the perimeter (lengths) must to be encompassed within three routes/sections, which all have rotational symmetry

by way of examples, here are some ways to transform four of the pentominoes
into 'trisides' :

can you see that each section (between the red dots) has rotational symmetry?

constructions:
start somewhere
try to use as much of the perimeter as you can
maybe with additional, extension, lines
to place a second dot so that this section has rotational symmetry
then try to place a third dot so that the next section has rotational symmetry

if the third section also has rotational symmetry then the resulting three dots form a triangle of area equal to the original shapes (5 squares in this case)

this is a 'triside' transformation for the original shape

trisides for a particular tessellating shape are not usually unique

for example, one pentomino:

where will the other two 'triside' corners/vertices be?

other pentominoes will also transform to 'trisides':
maybe attempt these:

by means of this transformation, tessellations of each pentomino can be seen to have a triangle tessellation lying 'behind' them


in trying to turn tessellating shapes into 'trisides', one technique is to start somewhere (A) and then use rotational symmetry to locate B from A and also C from A
then find C from B
adjusting the outcomes so that all three sections between the dots have rotational symmetry

if you end up with the third dot not in the same place as another but in line with it, that is good because going half way between them enables a 'triside' to be created:

furthermore, it seems that many straight line bounded shapes that tessellate can be turned into 'trisides'

sometimes with a little bit of perseverance...

where will the other two corners of a 'triside' be?

a chevron (hexagon) turned into a 'triside'

maybe also consider how the triangle can be turned into the chevron by cutting and fitting












a regular hexagon turned into a 'triside':

how can you see that the areas will be the same?

so,
are all tessellations of straight line bounded shapes
actually just tessellations of triangles?

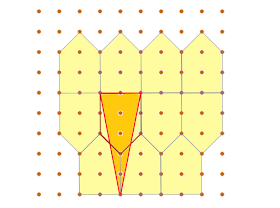
e.g. for one of the pentagons ('house') that will tessellate:

sometimes, for a shape that is difficult to 'triside'
it can be helpful to form a 'quadriside' as an intermediate step and then change this quadrilateral into a 'triside'

for example:

first form a 'quadriside'

then transform the quadrilateral into a 'triside'

another example of transforming a tessellating shape into a 'triside' via a 'quadriside':

you might also want to check that this shape does tessellate

sometimes the construction lines are difficult
where do the other two points of a 'triside' for this (tessellating) shape go?

tessellation of a 7-sided shape:

a 'triside' for this heptagon:

the arrow tessellation with the tessellation of 'trisides' as a basis for it:

one of the types of pentagon that will tessellate has two sides parallel
[the 'house' pentagon above is another example of this shape]

an associated 'triside' for these (generality of) pentagons:

some tessellating shapes are difficult (if not impossible) to find 'trisides' for
but where this is the case it can be possible to find a 'triside' for a pair of the shapes

two examples:

an example of a 'Cairo' pentagon tiling:

two of the pentagons form a 'unit' for a tessellation

you can find a 'triside' for this pair












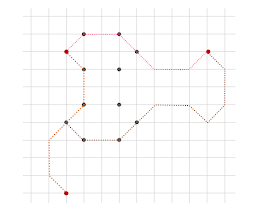
for this 12 sided polygon:

that can tessellate like this

a pair form a new tessellating unit

and you can find a 'triside' for this pair

one of the semi-regular tessellations (at least) can be turned into a 'triside'

so, I'm growing in my conviction that every tessellation of polygons has a triangle tessellation as a basis for the tessellation