'Cairo' pentagon tilings

a tessellation of a single symmetric pentagon,
can have (several) equal sides,
two (opposite) right angles

these come in various forms (have degrees of freedom)

found in Cairo as paving tiles
(not that old)

what relationship is there between the angles if two are right angles and two of the other (obtuse) angles are equal?

what if the 3 obtuse angles are equal?

David Bailey (from Grimsby, England, with a keen interest in recreational mathematics) has undertaken a very thorough analysis of the variety and dates of 'in situ' tiles
so far the oldest dated version he has been able to establish is 1956

due to the two 90 degree angles in the pentagon
the tessellation(s) have a 'skeleton' (as David Wells calls them) of squares

so the variety of this type of tessellation is created by the different angles in the rhombus:

the pentagon tessellation can be viewed with other square 'skeletons'
















or another way
are these squares?

or with isosceles (at least) triangles


what relationship is there for the apex and two equal base angles?














or with trapeziums
what is the relationship here?

















a version of the tiling can be created from the 4, 3, 3, 4, 3 semi-regular tessellation, as a dual (corners of the original tessellation become centres of the related one - and vice versus)

what are the angles in the tiles?

are the tiles congruent?

a tessellation of Cairo-like tiles can be drawn on isometric paper

but...  there are two different types of (non-congruent) pentagon here
establish that their angles are the same

David Bailey quotes Robert H Macmillan's claim that collinearity is a feature of some of the 'Cairo' tessellations and explores this

four of the sides are the same length

what are the angles in each pentagon tile for this arrangement?

use trigonometry to establish the angles in each pentagon for this arrangement

what collinearity is there? 

it is possible to have
all the sides the same length


















what are the angles for this equilateral pentagon tile?















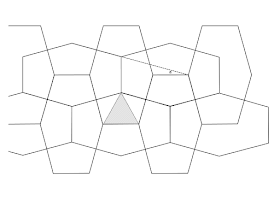
if the triangle formed by joining the apex of the pentagon to the two bottom corners is equilateral

there is a collinearity

the triangle shown is equilateral

find angle 'a'
and establish the (seeming) collinearity property